youngs modulus from compressive test|young's modulus definition : supermarket Young's modulus (or Young modulus) is a mechanical property of solid materials that measures the tensile or compressive stiffness when the force is applied lengthwise. It is the modulus of elasticity for tension or axial compression. Young's modulus is defined as the ratio of the stress (force per unit area) applied to the object and the resulting axial strain (displacement or deformation) in the linear elastic Resultado da 19 de jan. de 2024 · Approaching colleagues for feedback should be done with care and respect. Here are some guidelines: – Be clear. Explain why you are seeking feedback. – Choose the right time. Don’t catch them at a bad time. – Be open and receptive.
{plog:ftitle_list}
Resultado da Nossas calcinhas absorventes são perfeitas para sua menstruação, incontinência urinária leve a moderada, secreção vaginal e para o período .
Young’s modulus (E) is the modulus of elasticity under tension or compression. In other words, it describes how stiff a material is or how readily it bends or stretches. Young’s modulus relates stress (force per unit area) to .
For compressive strains, if we define \(\delta l=l_{0}-l>0\) then Equation \ref{26.2.3} holds for compressive stresses provided the compressive stress is not too large. For many materials, Young’s Modulus is the same . How to Calculate Young's Modulus from Unconfined Compression Test - YouTube. 0:00 / 7:19. Young's modulus (or modulus elasticity) is an important characteristic of rock which is.
Young's modulus (or Young modulus) is a mechanical property of solid materials that measures the tensile or compressive stiffness when the force is applied lengthwise. It is the modulus of elasticity for tension or axial compression. Young's modulus is defined as the ratio of the stress (force per unit area) applied to the object and the resulting axial strain (displacement or deformation) in the linear elastic
With this Young's modulus calculator, you can obtain the modulus of elasticity of a material, given the strain produced by a known tensile/compressive stress. We will also .Young’s modulus \(Y\) is the elastic modulus when deformation is caused by either tensile or compressive stress, and is defined by Equation \ref{12.33}. Dividing this equation by tensile .The compressive strength of the material corresponds to the stress at the red point shown on the curve. In a compression test, there is a linear region where the material follows Hooke's law. Hence, for this region, where, this time, E .
The ratio of stress to strain is called the elastic modulus. Hooke’s Law only holds for a range of stresses, a range referred to as the elastic region. An elastic body is one in which Hooke’s .Overview. The objectives of this practical are. 1. to demonstrate Hooke's law; 2. to determine the Young's modulus and Poisson's ratio of a material; 3. to determine the shear modulus of the .Young's Modulus, Tensile Strength and Yield Strength Values common Materials; Material Tensile Modulus (Young's Modulus, Modulus of Elasticity) - E - (GPa) Ultimate Tensile Strength - σ u-(MPa) Yield Strength - σ y-(MPa) .
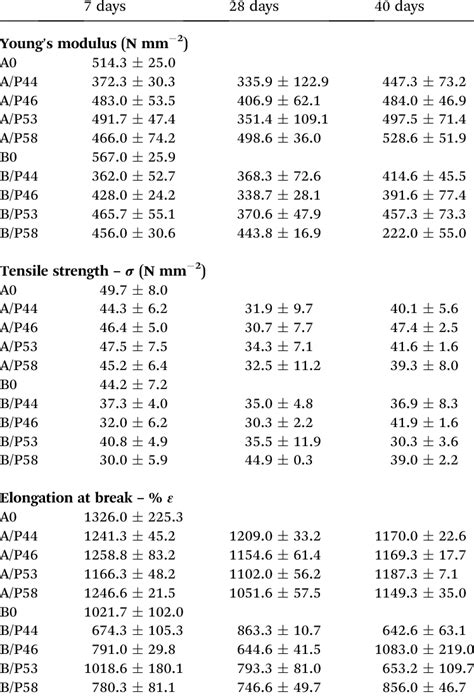
Where σ: Compressive Stress, P: Load and A 0: The initial cross-section area of the specimen. Therefore, the Unconfined Compressive Strength is calculated for the maximum load applied: σ UCS = P max / A 0. The modulus of elasticity .Materials with high Young's modulus are stiffer and less prone to deformation, while those with low Young's modulus are more flexible and easily deformable. This property is particularly important in applications where materials need to maintain their shape and resist deformation, such as in the design of springs, beams, and other load-bearing .In the early (low strain) portion of the curve, many materials obey Hooke’s law to a reasonable approximation, so that stress is proportional to strain with the constant of proportionality being the modulus of elasticity or Young’s modulus, denoted \(E\): \[\sigma_e = E_{\epsilon_e}\]Young’s modulus (E)- Ratio of tensile or compressive stress to the corresponding strain below the maximum stress a material can withstand without deviation from proportionality of stress to strain (proportional limit).Note that Young’s modulus in tension is different from Young’s modulus in compression. Test Procedure. A simplified test procedure includes the steps .
The new version of Hooke’s law is . Now we have , which is called Young’s Modulus or the modulus of elasticity.Young’s modulus provides the linear relationship between stress and strain. Young’s modulus is the same for any material–you could take a spoon or a girder; as long as they have the same young’s modulus and you knew their sizes, you could . Is the Young's Modulus different for Compressive and Tensile Stress? Hot Network Questions Is using online interaction platforms like Wooclap effective in a university math classroom?
young's modulus values table
In this paper, polymeric specimens are produced via the Fused Deposition Modelling (FDM) technique. Then, experimental tensile and compression tests are conducted to evaluate the main mechanical properties of elements made of PolyLacticAcid (PLA) material. A standardized characterization test method for FDM 3D printed polymers has not been . "modulus of compression" published on by null. The ratio of mechanical stress to strain in an elastic material when that material is being compressed; it is the modulus of elasticity applied to a material under compression:modulus of compression = compressive force per unit area/change in volume per unit volume. Young’s modulus (E) is the ratio of principal stress in one direction (σ x) to corresponding strain in the elastic range in the same direction (ε x) (ASTM 2014); it is named after the individual who identified it as an important material property, Thomas Young (1773–1829).It is also known as the elastic modulus. A plot of stress and strain from a laboratory uniaxial . Young’s modulus of intact rock ( $${E}_{i}$$ ) has a theoretical background influence on the deformation modulus of the rock mass ( $${D}_{f}$$ ); it is a critical input parameter in terms of the analytical and numerical stability analysis methods of underground spaces. . part of the stress–strain curve in the uniaxial compressive test of .
large gauge compression tester
Measurement of the elastic moduli of a brick-shaped gelatin dessert gel piece of top area A.(a) Gel deformation under a compressive force (F c): the height of the gel decreases from H o to H while its width increases from W o to W.The normal stress and strain of the gel are σ = F c /A and ε = (H o − H)/H o, respectively.(b) Gel deformation under a tensile force (F t): .The elastic modulus of an object is defined as the slope of its stress–strain curve in the elastic deformation region: [1] A stiffer material will have a higher elastic modulus. An elastic modulus has the form: = where stress is the force causing the deformation divided by the area to which the force is applied and strain is the ratio of the change in some parameter caused by the .
However, the concept of Young’s modulus of elasticity is frequently used to express the stress-strain ratio of the food, at least under moderately light compressions and in the area of the force-compression curve that is reasonably linear. . Properties that can be measured with a compression test. Compression tests are typically chosen to .
larson spring tester dht 11 lb compression digital hand tester
young's modulus theory
We would like to show you a description here but the site won’t allow us.It generally refers to the static Young's modulus, which can be obtained from laboratory core tests, e.g., uniaxial or triaxial compression test. The dynamic Young's modulus can be calculated from theoretical equations using the acoustic velocity data. However, for geomechanics analysis, static Young's modulus is needed. Young's modulus (or modulus elasticity) is an important characteristic of rock which is used in design as it provides estimates of rock deformations under lo.

E = Young's Modulus (N/m 2) (lb/in 2, psi) Modulus of Elasticity, or Young's Modulus, is commonly used for metals and metal alloys and expressed in terms 10 6 lb f /in 2, N/m 2 or Pa. Tensile modulus is often used for plastics and is expressed in terms 10 5 lb f /in 2 or GPa. Shear Modulus of Elasticity - or Modulus of Rigidity. G = stress .
Technical Note: Determination of Young's Modulus and Poisson's Ratio for Intact Stratified Rocks and their Relationship with Uniaxial Compressive Strength 5.3 Since for many materials, Young's modulus in tension is different from Young's modulus in compression, it shall be derived from test data obtained in the stress mode of interest. 5.4 The accuracy and precision of apparatus, test specimens, and procedural steps should be such as to conform to the material being tested and to a reference . As it has already been pointed out in Section 4.1, the Young's modulus of the material can be obtained from an indentation test as long as the value of the Poisson's Discussion The range of porosity ratios considered in the present study (45–67%) is relatively high if compared to those of porous rocks, seldom greater than 30%.
Discover material stiffness with the Young's Modulus Calculator. Measure the squishiness or rigidity for any material! . Complex test setup for accurate results: Moderate: Evolution of Young’s Modulus Calculation: Year . Young’s Modulus measures stiffness under tensile or compressive loads, while Shear Modulus measures stiffness under .
The laboratory test to determine the modulus of elasticity of concrete is explained below. Procedure. The test procedure involves two stages. Initially, the compressometer is set-up, followed by the application of load and testing. . Setting Up Compressometer. A compressometer is a device used in the compression test of the concrete cylinder .The uniaxial compressive strength and static Young's modulus (Es) of intact rocks are the most important geotechnical parameters for stability analysis of surface and underground structures.
A series of experiments and numerical simulations based on uniaxial compression test have been carried out over the past decades to study the mechanical failures (Zhang and . An adaptive neuro-fuzzy inference system for predicting unconfined compressive strength and Young's modulus: a study on Main Range granite. Bull. Eng. Geol. Environ., 74Tensile modulus and Young's modulus are both mechanical properties used to measure the sti. This vs. That Explore Comparisons. vs. . Young's modulus measures the ratio of stress to strain in a material under any type of stress, including tensile, compressive, or shear stress. In essence, Young's modulus provides a more general measure of a . The uniaxial compressive strength values obtained from both types of test show a very good agreement. For the Young's modulus, the values obtained from the two types of test are different but the variation of the moduli with porosity is the same. . (uniaxial compressive strength and Young's modulus). Wilson et al. (1975) .
young's modulus definition
3 de mar. de 2023 · Para assistir Hercai em Português - Capítulo 43: 👉 https://youtu.be/3Ue-i2mmsSgPara se inscrever no canal do YouTube de Eu Gosto de Séries Turcas: 👉 https:.
youngs modulus from compressive test|young's modulus definition